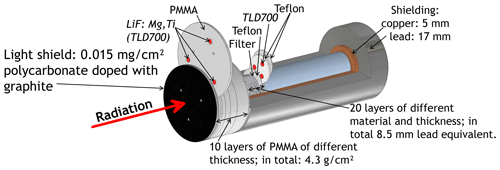
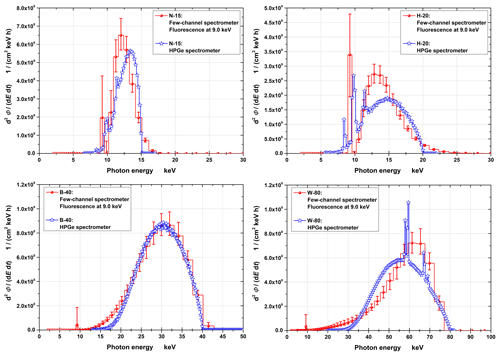
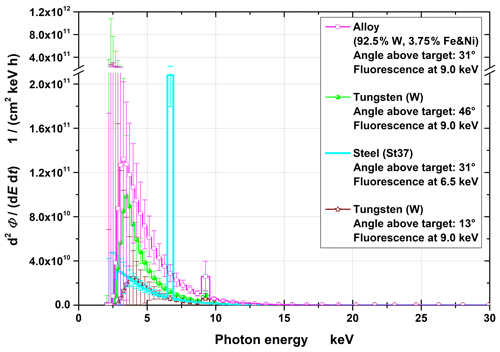
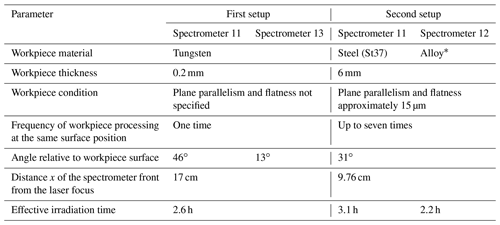
Ankerhold, U.: Catalogue of X-ray spectra and their characteristic data – ISO
and DIN radiation qualities, therapy and diagnostic radiation qualities,
unfiltered X-ray spectra, PTB report Dos-34, https://doi.org/10.7795/110.20190315B, 2000.
Ankerhold, U., Behrens, R., and Ambrosi, P.: X-ray spectrometry of low
energy photons for determining conversion coefficients from air kerma,
Ka, to personal dose equivalent, Hp(10), for radiation qualities of
the ISO narrow spectrum series, Radiat. Prot. Dosim., 81, 247
https://doi.org/10.1093/oxfordjournals.rpd.a032591, 1999.
Behrens, R.: A spectrometer for pulsed and continuous photon radiation, J.
Instrum., 4, P03027, https://doi.org/10.1088/1748-0221/4/03/P03027, 2009.
Behrens, R.: Conversion coefficients for H′(3;Ω) for photons, J.
Radiol. Prot., 37, 354, https://doi.org/10.1088/1361-6498/aa51e8, 2017.
Behrens, R. and Ambrosi, P.: A TLD-based Few-channel Spectrometer for
Mixed Photon, Electron, and Ion Fields with High Fluence Rates, Radiat.
Prot. Dosim. 101, 73, https://doi.org/10.1093/oxfordjournals.rpd.a006062, 2002.
Behrens, R. and Reginatto, M.: Bayesian Spectrum Deconvolution Including
Uncertainties and Model Selection: Application to X-Ray Emission Data
Using WinBUGS, Radiat. Prot. Dosim., 185, 157–167, https://doi.org/10.1093/rpd/ncy286,
2019.
Behrens, R., Pullner, B., and Reginatto, M.: X-Ray Emission from Materials
Processing Lasers, Radiat. Prot. Dosim. 183, 361–374,
https://doi.org/10.1093/rpd/ncy126, 2019.
Bielajew, A. and Rogers, D. W. O.: PRESTA: The Parameter Reduced Electron-Step
Transport Algorithm for Electron Monte Carlo Transport, Nucl. Instrum.
Methods, 18, 165, https://doi.org/10.1016/S0168-583X(86)80027-1, 1987.
Gerhard, C., Viöl, W., and Wieneke, S.: Plasma-Enhanced Laser Materials
Processing, Plasma Science and Technology, Progress in Physical States and
Chemical Reactions, Tetsu Mieno, IntechOpen, London, available at: https://cdn.intechopen.com/pdfs/49531.pdf (last access: 16 February 2021), 2016.
International Commission on Radiation Units and Measurements (ICRU):
Conversion Coefficients for use in Radiological Protection against External
Radiation, ICRU Report 57, ICRU, Bethesda, https://doi.org/10.1093/jicru/os29.2.Report57, 1998.
International Organization for Standardization (ISO): Radiological
protection, X and gamma reference radiation for calibrating dosemeters and
doserate meters and for determining their response as a function of photon
energy – Part 1: Radiation characteristics and production methods,
ISO 4037-1, 2019.
Lunn, D. J., Thomas, A., Best, N., and Spiegelhalter, D.: WinBUGS – a
Bayesian modelling framework: Concepts, structure, and extensibility,
Stat. Comput., 10, 325, https://doi.org/10.1023/A:1008929526011, 2010.
Momma, C., Chichkova, B. N., Nolte, S., von Alvensleben, F., Tünnermann, A., Welling, H., and Wellegehausen, B.: Short-pulse laser ablation of solid targets, Opt. Commun.,
129, 134, http://dx.doi.org/10.1016/0030-4018(96)00250-7, 1996.
Mulser, P. and Bauer, D.: High Power Laser-Matter Interaction,
Springer, Berlin and Heidelberg, Germany, https://doi.org/10.1007/978-3-540-46065-7,
2010.
Namito, Y., Ban, S., and Hirayama, H.: LSCAT: Low-Energy Photon-Scattering
Expansion for the EGS4 Code, National Laboratory for High Energy Physics,
Report KEK Internal 95-10, 51 pp., Tsukuba, Japan, 1995.
Nelson, W. R., Hirayama, H., and Rogers, D. W. O.: The EGS4 Code System, Report SLAC-265,
Stanford Linear Accelerator Center, 398 pp., Virginia, 1985.
Nolte, S., Schrempel, F., and Dausinger, F.: Ultrashort pulse laser
technology, Laser sources and applications, Springer, Cham, Heidelberg, New York, Dordrecht, London, https://doi.org/10.1007/978-3-319-17659-8, 2016.
StrlSchG: Radiation Protection Act of 27 June 2017,
(BGBl. 1966), as last amended by Article 5 of the Act of 23 October 2020 (BGBl. 2232), 2017.
StrlSchV: Radiation Protection Ordinance of 29 November 2018, (BGBl.
2034, 2036), as last amended by Article 1 of the Ordinance of 20 November
2020 (BGBl. 2502), 2018.
WinBUGS: WinBUGS code, University of Cambridge, MRC Biostatistics Unit, available at: https://www.mrc-bsu.cam.ac.uk/software/bugs/the-bugs-project-winbugs/, last acess: 16 February 2021.